Engineering a structure involves not only dimensioning the structure but also evaluating whether the structure’s response under the construction and design loadings satisfy the specified design criteria. Response evaluation is commonly referred to as structural analysis and is carried out with certain analytical methods developed in the field of Engineering Mechanics and adopted for structural systems. In this section, we review these methods and illustrate their application to some simple structures.

Most of this material is covered in textbooks dealing with Statics and Mechanics of Materials and Structural Analysis. Heyman’s text contains an excellent description of the “underlying science of Structural Engineering.”

Concept of Equilibrium-Concurrent Force System

We begin with a discussion of equilibrium conditions for solid bodies. This topic is relevant to structural engineering since structures are solid bodies subjected to loads, and we need to ensure that a structure remain at rest, i.e., that it is in a state of equilibrium.

The simplest case is a body subjected to a set of concurrent forces. By definition, the lines of action of the forces comprising a concurrent force system intersect at a common point. Figure 1.21 illustrates this case. For static equilibrium, the resultant of the force system must be a null vector

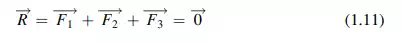
We convert the vector equilibrium over to a set of algebraic equations by resolving the force vectors into their components with respect to an arbitrary set of orthogonal directions (X, Y, Z). This operation leads to

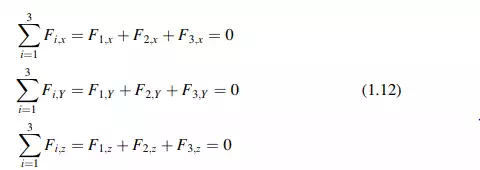
We find it more convenient to work with (1.12) rather than (1.11).

When all the force vectors are in one plane, say the X–Y plane, the force system is called a planar force system and (1.12) reduces to 2 equations. Most of the force systems that we deal with will be planar systems.

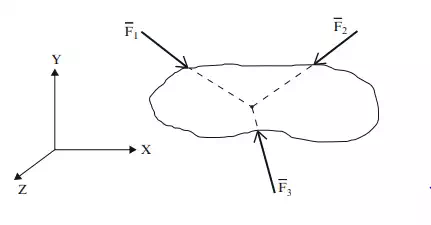
Fig. 1.21 Concurrent force system

Concept of Equilibrium: Non-concurrent Force System

The next level of complexity is a body subjected to a non-concurrent planar force system. Referring to Fig. 1.22, the forces tend to rotate the body as well as translate it. Static equilibrium requires the resultant force vector to vanish and, in addition, the resultant moment vector about an arbitrary point to vanish.

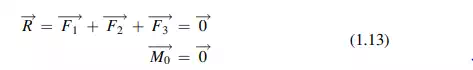
Resolving the force and moment vectors into their X, Y, Z components leads to six scalar equations, three for force and three for moment.

When the force system is planar, say in the X–Y plane, the equations (1.13) reduce to three scalar equations

where O is an arbitrary point in the x–y plane. Note that now for a planar system there are three equilibrium conditions vs. two for a concurrent system. Note also that since there are three equilibrium equations, one needs to apply three restraints to prevent planar rigid body motion

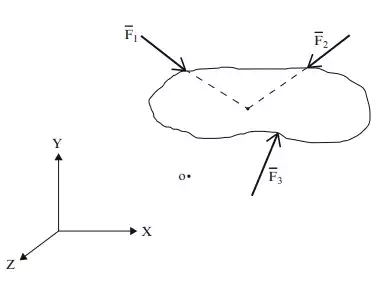
Fig. 1.22 Non-concurrent force system